- 您現(xiàn)在的位置：買賣IC網(wǎng) > PDF目錄373974 > ADA4938-1_07 (Analog Devices, Inc.) Ultra-Low Distortion Differential ADC Driver PDF資料下載
參數(shù)資料
| 型號： | ADA4938-1_07 |
| 廠商： | Analog Devices, Inc. |
| 英文描述： | Ultra-Low Distortion Differential ADC Driver |
| 中文描述： | 超低失真差分ADC驅(qū)動(dòng)器 |
| 文件頁數(shù)： | 10/14頁 |
| 文件大?。?/td> | 307K |
| 代理商： | ADA4938-1_07 |
ADA4938-1
Preliminary Technical Data
THEORY OF OPERATION
The ADA4938-1 differs from conventional op amps in that it
has two outputs whose voltages move in opposite directions.
Like an op amp, it relies on open-loop gain and negative
feedback to force these outputs to the desired voltages. The
ADA4938-1 behaves much like a standard voltage feedback op
amp and makes it easier to perform single-ended-to-differential
conversions, common-mode level shifting, and amplifications
of differential signals. Also like an op amp, the ADA4938-1 has
high input impedance and low output impedance.
Two feedback loops are employed to control the differential and
common-mode output voltages. The differential feedback, set
with external resistors, controls only the differential output
voltage. The common-mode feedback controls only the common-
mode output voltage. This architecture makes it easy to set the
output common-mode level to any arbitrary value. It is forced,
by internal common-mode feedback, to be equal to the voltage
applied to the V
OCM
input, without affecting the differential
output voltage.
The ADA4938-1 architecture results in outputs that are highly
balanced over a wide frequency range without requiring tightly
matched external components. The common-mode feedback
loop forces the signal component of the output common-
mode voltage to zero. This results in nearly perfectly balanced
differential outputs that are identical in amplitude and are
exactly 180° apart in phase.
ANALYZING AN APPLICATION CIRCUIT
The ADA4938-1 uses open-loop gain and negative feedback to
force its differential and common-mode output voltages in such
a way as to minimize the differential and common-mode error
voltages. The differential error voltage is defined as the voltage
between the differential inputs labeled +IN and IN (see
Figure 4). For most purposes, this voltage can be assumed to be
zero. Similarly, the difference between the actual output
common-mode voltage and the voltage applied to V
OCM
can also
be assumed to be zero. Starting from these two assumptions,
any application circuit can be analyzed.
Rev. PrD | Page 10 of 14
SETTING THE CLOSED-LOOP GAIN
The differential-mode gain of the circuit in Figure 4 can be
determined by
G
F
dm
IN
dm
OUT
V
R
R
V
=
,
,
This assumes the input resistors (
R
G
) and feedback resistors (
R
F
)
on each side are equal.
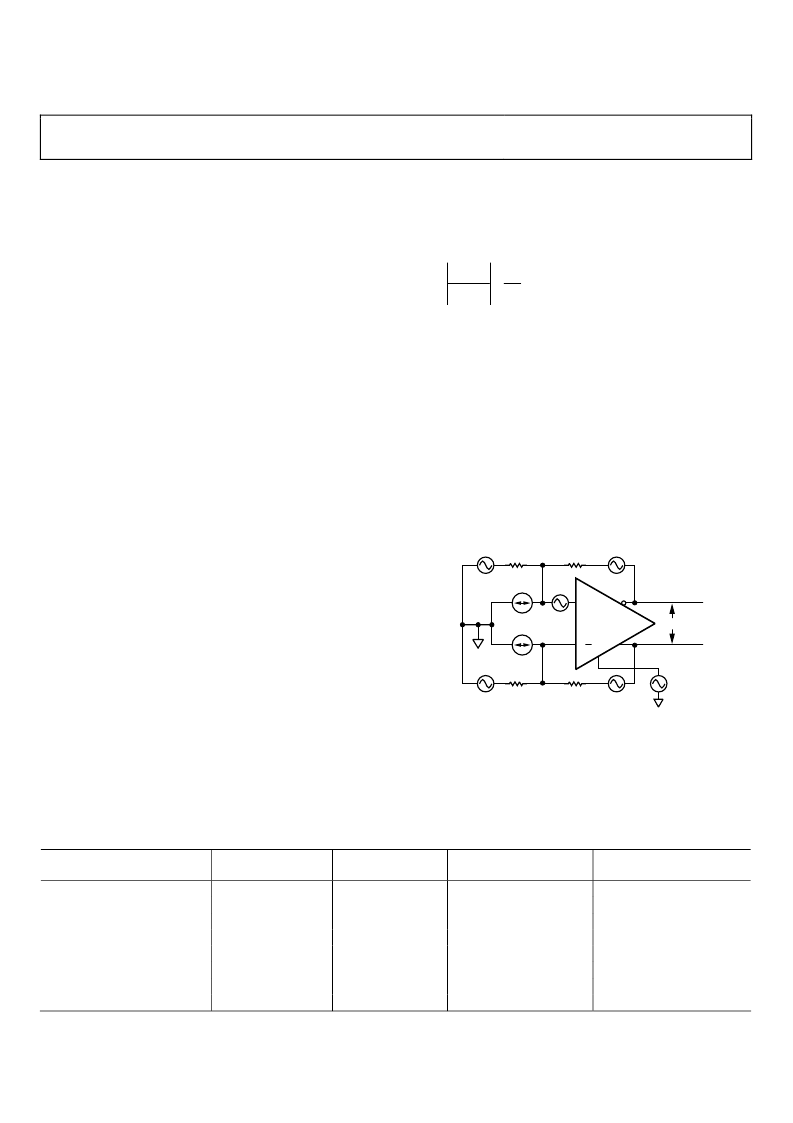
ESTIMATING THE OUTPUT NOISE VOLTAGE
The differential output noise of the ADA4938-1 can be
estimated using the noise model in Figure 5. The input-referred
noise voltage density, v
nIN
, is modeled as a differential input, and
the noise currents, i
nIN
and i
nIN+
, appear between each input and
ground. The noise currents are assumed to be equal and
produce a voltage across the parallel combination of the gain
and feedback resistances. v
nCM
is the noise voltage density at the
V
OCM
pin. Each of the four resistors contributes (4kTR
x
)
1/2
. Table
6 summarizes the input noise sources, the multiplication factors,
and the output-referred noise density terms.
ADA4938-1
+
R
F2
V
nOD
V
nCM
V
OCM
V
nIN
R
F1
R
G2
R
G1
V
nRF1
V
nRF2
V
nRG1
V
nRG2
i
nIN+
i
nIN–
Figure 5. ADA4938-1 Noise Model
Table 6. Output Noise Voltage Density Calculations
Input Noise Contribution
Differential Input
Inverting Input
Noninverting Input
V
OCM
Input
Gain Resistor R
G1
Gain Resistor R
G2
Feedback Resistor R
F1
Feedback Resistor R
F2
Input Noise Term
v
nIN
i
nIN
i
nIN+
v
nCM
v
nRG1
v
nRG2
v
nRF1
v
nRF2
Input Noise
Voltage Density
v
nIN
i
nIN
× (R
G2
||R
F2
)
i
nIN+
× (R
G1
||R
F1
)
v
nCM
(4kTR
G1
)
1/2
(4kTR
G2
)
1/2
(4kTR
F1
)
1/2
(4kTR
F2
)
1/2
Output
Multiplication Factor
G
N
G
N
G
N
G
N
(β
1
β
2
)
G
N
(1 β
2
)
G
N
(1 β
1
)
1
1
Output Noise
Voltage Density Term
v
nO1
= G
N
(v
nIN
)
v
nO2
= G
N
[i
nIN
× (R
G2
||R
F2
)]
v
nO3
= G
N
[i
nIN+
× (R
G1
||R
F1
)]
v
nO4
= G
N
(β
1
β
2
)(v
nCM
)
v
nO5
= G
N
(1 β
2
)(4kTR
G1
)
1/2
v
nO6
= G
N
(1 β
1
)(4kTR
G2
)
1/2
v
nO7
= (4kTR
F1
)
1/2
v
nO8
= (4kTR
F2
)
1/2
相關(guān)PDF資料 |
PDF描述 |
|---|---|
| ADA4938-1ACPZ-R2 | Ultra-Low Distortion Differential ADC Driver |
| ADA4938-1ACPZ-R7 | Ultra-Low Distortion Differential ADC Driver |
| ADA4938-1ACPZ-RL | Ultra-Low Distortion Differential ADC Driver |
| ADA4938-1 | Ultra-Low Distortion Differential ADC Driver |
| ADA4938-1_06 | Ultra-Low Distortion Differential ADC Driver |
相關(guān)代理商/技術(shù)參數(shù) |
參數(shù)描述 |
|---|---|
| ADA4938-1ACPZ-R2 | 制造商:Analog Devices 功能描述:SP Amp DIFF AMP Single ±5.5V/11V 16-Pin LFCSP EP T/R 制造商:Analog Devices 功能描述:SP AMP DIFF AMP SGL 5.5V/11V 16LFCSP EP - Tape and Reel 制造商:Analog Devices 功能描述:SP Amp DIFF AMP Single }5.5V/11V 16-Pin LFCSP EP Tape & Reel 制造商:Analog Devices 功能描述:IC AMP DIFFADCDR16BIT16LFCSP |
| ADA4938-1ACPZ-R2 | 制造商:Analog Devices 功能描述:IC DIFF AMP 1GHZ 4700V/S LFCSP-16 |
| ADA4938-1ACPZ-R7 | 功能描述:IC AMP DIFF ADC DR 16BIT 16LFCSP RoHS:是 類別:集成電路 (IC) >> 線性 - 放大器 - 專用 系列:- 產(chǎn)品培訓(xùn)模塊:Lead (SnPb) Finish for COTS Obsolescence Mitigation Program 標(biāo)準(zhǔn)包裝:60 系列:- 類型:可變增益放大器 應(yīng)用:CATV 安裝類型:表面貼裝 封裝/外殼:20-WQFN 裸露焊盤 供應(yīng)商設(shè)備封裝:20-TQFN-EP（5x5） 包裝:托盤 |
| ADA4938-1ACPZ-RL | 功能描述:IC ADC DRIVER DIFF 16-LFCSP RoHS:是 類別:集成電路 (IC) >> 線性 - 放大器 - 專用 系列:- 產(chǎn)品培訓(xùn)模塊:Lead (SnPb) Finish for COTS Obsolescence Mitigation Program 標(biāo)準(zhǔn)包裝:60 系列:- 類型:可變增益放大器 應(yīng)用:CATV 安裝類型:表面貼裝 封裝/外殼:20-WQFN 裸露焊盤 供應(yīng)商設(shè)備封裝:20-TQFN-EP（5x5） 包裝:托盤 |
| ADA4938-1YCP-EBZ | 功能描述:BOARD EVAL FOR ADA4938-1YCP RoHS:是 類別:編程器，開發(fā)系統(tǒng) >> 評估板 - 運(yùn)算放大器 系列:- 產(chǎn)品培訓(xùn)模塊:Lead (SnPb) Finish for COTS Obsolescence Mitigation Program 標(biāo)準(zhǔn)包裝:1 系列:- |
發(fā)布緊急采購，3分鐘左右您將得到回復(fù)。