- 您現(xiàn)在的位置:買賣IC網(wǎng) > PDF目錄385491 > LMC6035IMMX (NATIONAL SEMICONDUCTOR CORP) Low Power 2.7V Single Supply CMOS Operational Amplifiers PDF資料下載
參數(shù)資料
| 型號(hào): | LMC6035IMMX |
| 廠商: | NATIONAL SEMICONDUCTOR CORP |
| 元件分類: | 運(yùn)動(dòng)控制電子 |
| 英文描述: | Low Power 2.7V Single Supply CMOS Operational Amplifiers |
| 中文描述: | DUAL OP-AMP, 5000 uV OFFSET-MAX, 1.4 MHz BAND WIDTH, PDSO8 |
| 封裝: | 0.150 INCH, MSOP-8 |
| 文件頁數(shù): | 12/20頁 |
| 文件大小: | 790K |
| 代理商: | LMC6035IMMX |
1.0 Application Notes
(Continued)
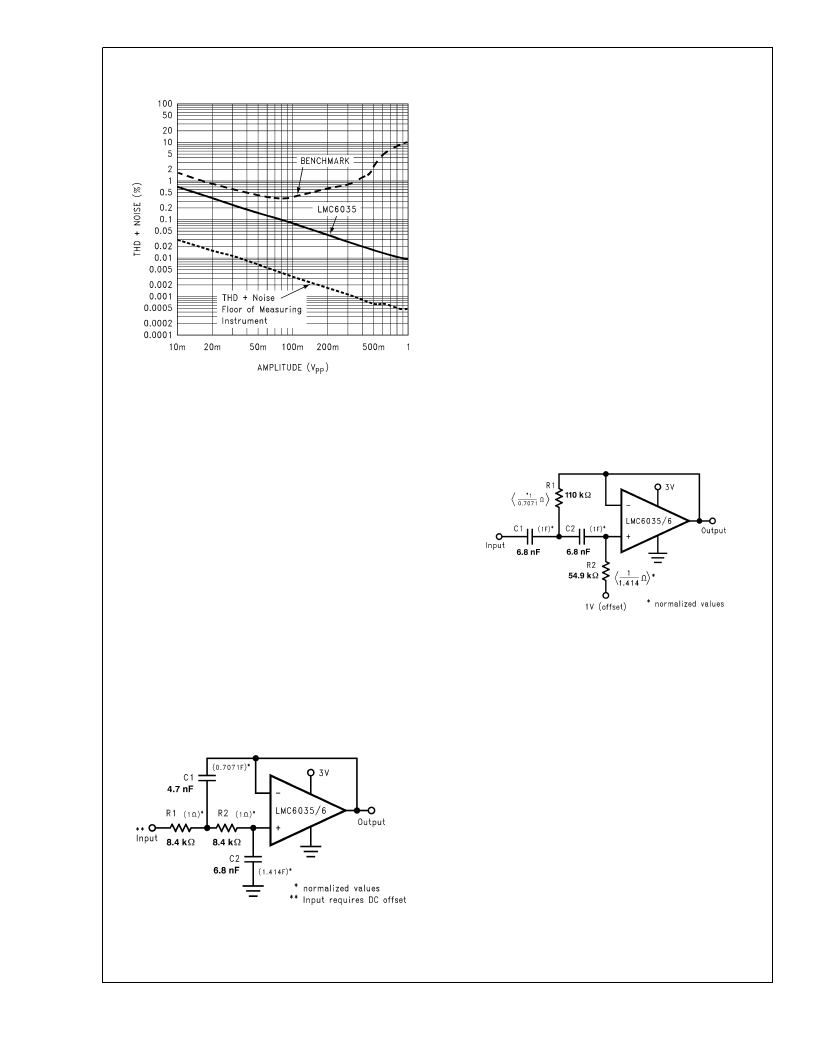
Figure 4 shows the superior distortion performance of
LMC6035/6 over that of the benchmark op amp. The heavy
loading of the circuit causes the A
of the benchmark part
to drop significantly which causes increased distortion.
1.2 APPLICATION CIRCUITS
1.2.1 Low-Pass Active Filter
A common application for low voltage systems would be ac-
tive filters, in cordless and cellular phones for example. The
ultra low input currents (I
) of the LMC6035/6 makes it well
suited for low power active filter applications, because it al-
lows the use of higher resistor values and lower capacitor
values. This reduces power consumption and space.
Figure 5 shows a low pass, active filter with a Butterworth
(maximally flat) frequency response. Its topology is a Sallen
and Key filter with unity gain. Note the normalized compo-
nent values in parenthesis which are obtainable from stan-
dard filter design handbooks. These values provide a 1 Hz
cutoff frequency, but they can be easily scaled for a desired
cutoff frequency (f
c
). The bold component values of Figure 5
provide a cutoff frequency of 3 kHz. An example of the scal-
ing procedure follows Figure 5
1.2.1.1 Low-Pass Frequency Scaling Procedure
The actual component values represented in bold of Figure 5
were obtained with the following scaling procedure:
1.
First determine the frequency scaling factor (FSF) for
the desired cutoff frequency. Choosing f
c
at 3 kHz, pro-
vides the following FSF computation:
FSF = 2
π
x 3 kHz
(desired cutoff freq.)
= 18.84 x 10
3
2.
Then divide all of the normalized capacitor values by the
FSF as follows:
C1’ = C
/FSF
C1’ = 0.707/18.84 x 10
3
= 37.93 x 10
6
C2’ = 1.414/18.84 x 10
3
= 75.05 x 10
6
(C1’ and C2’: prior to impedance scaling)
3.
Last, choose an impedance scaling factor (Z). This Z
factor can be calculated from a standard value for C2.
Then Z can be used to determine the remaining compo-
nent values as follows:
Z = C2’/C2
(chosen)
= 75.05 x 10
6
/
6.8 nF
= 8.4k
C1 = C1’/Z = 37.93 x 10
6
/8.4k = 4.52 nF
(Standard capacitor value chosen for C1 is
4.7 nF
)
R1 = R1
(normalized)
x Z = 1
x 8.4k = 8.4 k
R2 = R2
(normalized)
x Z = 1
x 8.4k = 8.4 k
(Standard value chosen for R1 and R2 is
8.45 k
)
1.2.2 High Pass Active Filter
The previous low-pass filter circuit of Figure 5 converts to a
high-pass active filter per Figure 6
1.2.2.1 High-Pass Frequency Scaling Procedure
Choose a standard capacitor value and scale the imped-
ances in the circuit according to the desired cutoff frequency
(300 Hz) as follows:
C = C1 = C2
Z = 1 Farad/C
(chosen)
x 2
π
x (desired cutoff freq.)
= 1 Farad/
6.8 nF
x 2
π
x 300 Hz = 78.05k
R1 = Z x R1
(normalized)
= 78.05k x (1/0.707) = 110.4 k
(Standard value chosen for R1 is
110 k
)
R2 = Z x R2
(normalized)
= 78.05k x (1/1.414) = 55.2 k
(Standard value chosen for R1 is
54.9 k
)
1.2.3 Dual Amplifier Bandpass Filter
The dual amplifier bandpass (DABP) filter features the ability
to independently adjust f
and Q. In most other bandpass to-
pologies, the f
and Q adjustments interact with each other.
The DABP filter also offers both low sensitivity to component
values and high Qs. The following application of Figure 7
provides a 1 kHz center frequency and a Q of 100.
DS012830-47
FIGURE 4. THD+Noise Performance of LMC6035 and
“Benchmark” per Circuit of
Figure 1
DS012830-48
FIGURE 5. 2-Pole, 3 kHz, Active, Sallen and Key,
Lowpass Filter with Butterworth Response
DS012830-49
FIGURE 6. 2 Pole, 300 Hz, Sallen and Key,
High-Pass Filter
L
www.national.com
12
相關(guān)PDF資料 |
PDF描述 |
|---|---|
| LMC6035ITL | Low Power 2.7V Single Supply CMOS Operational Amplifiers |
| LMC6035ITLX | Low Power 2.7V Single Supply CMOS Operational Amplifiers |
| LMC6042 | CMOS Dual Micropower Operational Amplifier |
| LMC6042AIM | CMOS Dual Micropower Operational Amplifier |
| LMC6042AIN | CMOS Dual Micropower Operational Amplifier |
相關(guān)代理商/技術(shù)參數(shù) |
參數(shù)描述 |
|---|---|
| LMC6035IMMX/NOPB | 功能描述:運(yùn)算放大器 - 運(yùn)放 RoHS:否 制造商:STMicroelectronics 通道數(shù)量:4 共模抑制比(最小值):63 dB 輸入補(bǔ)償電壓:1 mV 輸入偏流(最大值):10 pA 工作電源電壓:2.7 V to 5.5 V 安裝風(fēng)格:SMD/SMT 封裝 / 箱體:QFN-16 轉(zhuǎn)換速度:0.89 V/us 關(guān)閉:No 輸出電流:55 mA 最大工作溫度:+ 125 C 封裝:Reel |
| LMC6035IMQ1 | 制造商:Texas Instruments 功能描述:IC OPAMP GP 1.4MHZ RRO 8SOIC 制造商:Texas Instruments 功能描述:Low Power 2.7V CMOS Op Amp |
| LMC6035IMX | 功能描述:運(yùn)算放大器 - 運(yùn)放 RoHS:否 制造商:STMicroelectronics 通道數(shù)量:4 共模抑制比(最小值):63 dB 輸入補(bǔ)償電壓:1 mV 輸入偏流(最大值):10 pA 工作電源電壓:2.7 V to 5.5 V 安裝風(fēng)格:SMD/SMT 封裝 / 箱體:QFN-16 轉(zhuǎn)換速度:0.89 V/us 關(guān)閉:No 輸出電流:55 mA 最大工作溫度:+ 125 C 封裝:Reel |
| LMC6035IMX/NOPB | 功能描述:運(yùn)算放大器 - 運(yùn)放 RoHS:否 制造商:STMicroelectronics 通道數(shù)量:4 共模抑制比(最小值):63 dB 輸入補(bǔ)償電壓:1 mV 輸入偏流(最大值):10 pA 工作電源電壓:2.7 V to 5.5 V 安裝風(fēng)格:SMD/SMT 封裝 / 箱體:QFN-16 轉(zhuǎn)換速度:0.89 V/us 關(guān)閉:No 輸出電流:55 mA 最大工作溫度:+ 125 C 封裝:Reel |
| LMC6035IMXQ1 | 制造商:Texas Instruments 功能描述:IC OPAMP GP 1.4MHZ RRO 8SOIC 制造商:Texas Instruments 功能描述:Low Power 2.7V CMOS Op Amp |
發(fā)布緊急采購,3分鐘左右您將得到回復(fù)。